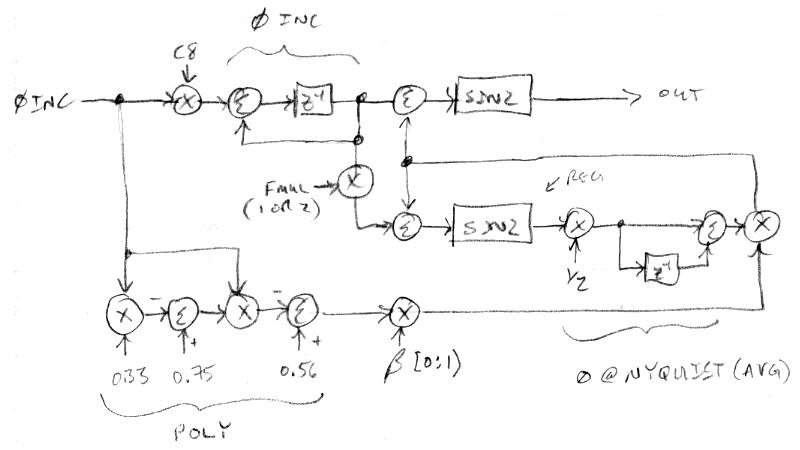
"Hmmm. Ok, so the DSP people with their current oscillator designs build upon some psycho-acoustic "tricks" to get a way with some things which we can't hear directly - but what if further signal processing then makes things behave differently than from what the whole system would behave like if it actually was alias free? Is it really equivalent to an "alias free oscillator" then? Like, if you sell an oscillator component, and don't know what exactly people are going to do with the signal (funky non linear stuff)... not sure how else to put it... Am I making sense?" - tinkeringdude
The more psycho-acoustic "trick" type approaches are low pass filtering (to reduce aliasing below the fundamental), comb filtering (to reduce aliasing between the harmonics) - both of these rely on masking (the ear being deaf to close frequencies that are lower in amplitude). Others mimic higher rate generation and downsampling, rather like multi-rate filters that don't calculate intermediate results which will ultimately be discarded. A couple of others, including the one I've finally picked, rely on secondary roll-off between the upper threshold of hearing and Nyquist to attenuate fold-back.
It's possible to do things like PWM and sync with base-band (i.e. non-oversampled) digital oscillators, I've read about this but haven't experimented along these lines.
I imagine the noise floor of an analog oscillator tends to white. While the digital noise floor could be anything, and I suspect "perfect repetition" over many cycles that digital processes often do can lead to audible "tells". And I imagine amplifying the noise floor could unmask aliasing in certain scenarios (though if they conform to something like the MP3 masking function like they are probably pretty insignificant).
"Ultrasonic, there you remind me of this weird thing, seems kinda Star Trek-ey, and the dev kit costs about as much as a "dilithium crystal", I imagine:
https://www.ultrahaptics.com/products-programs/touch-development-kit/"
~$5k USD, eek! It must require a lot of power for one's fingers to feel the ultrasonics?
Reminds me of the "perfect speaker" from a couple of decades ago, where they heterodyned ultrasonics in the air with enough power to make the medium go non-linear. It supposedly gives you private and localized audio sources, but I wonder what it does to the rest of the body long-term.
Easy, but only because there is an equivocation at play here
In analog synth world, when people say "VCF", they have certain ideas of what it does, and clinical filtering is only one aspect, kinda like literally amplifying is only a part of what an electric guitar's amp + speaker are about.
Have you used an analog, say MiniMoog ladder filter? And tortured it a bit?
(as for what it generally does, I guess you have seen the paper by Tim Stinchcombe? I can't tell how comprehensively it captures what's going on in what signal scenarios, too much greek, probably literally, heh)
I see your points and very much appreciate them! And I'll look at your links a bit later (the SO is hosting two piano recitals today and I'm the unhired hand). I haven't played around at all with modern analog synth filters, though long ago I built a second order state-variable as a guitar effect, and the digital filter in our AN-1x was really fun to control with the ribbon.
Forcing a digital filter to do non-linear things would almost certainly cause aliasing, and perhaps this is the main reason they went with analog filters.
"This editor is... not fully cooperative..."
Yes, I've fallen back to copying and pasting from an external editor to get around all the unwanted formatting insertion. There is a "reveal codes" button all the way to the right that allows one to clean up the remaining formatting dregs. And drag & drop seems to work better than copy & paste.
I have to do this in Word all the time too, "Paste special..." "unformatted text" should be the default (but alas it isn't). Word holds onto extraneous formatting like a Pit Bull on a steak...
[EDIT] OK, had some time this morning to look into this a bit and think about it. I must say, the Moog ladder filter is one of the stranger analog circuits I've encountered, though I first saw a simpler version of the diode ladder in the PAIA low pass VCF schematic. Not sure who invented it first, but it seems every synth designer more or less copied everyone else with just enough slight variation to avoid legal infringement / hype the difference. I think I first saw it in this paper by Stinchcombe (link) which led me to this paper by Stilson & Smith (link) - digitizing the linear response is non-trivial, and digitizing the non-linear response probably even more problematic. It's interesting that tanh shows up in both the analog analysis and in the paper I pointed to recently using tanh digitally to generate controlled harmonics.
It's kind of weird to me that simple analog synthesis is a "thing" in terms of sound. I mean, everyone likes to play with knobs, and interactive filtering can be loads of fun, but the basic waveforms are really dull or harsh sounding to me, without a lot of in-between. I suppose I've come to appreciate variable harmonic content not gotten by way of filtering, but by way of generation. Don't get me wrong, I love electronica, ambient, dance music, etc. but I don't think what remains of my life would be any less rich if I never spent any more time playing with an analog synth or the DSP equivalent or some combination. The thrill for me is mostly gone (and quite frankly was never all that big to begin with).
There are some things that are easy to do in analog that require a lot of study and effort to do in DSP, though with processors becoming almost free that trade-off is becoming more and more lopsided. And there are some thing that are easy to do in DSP that are virtually impossible to do in analog (delay, stability, low noise floor, tons of virtual knobs, ease of manufacture, ease of complexity, etc.). It's too bad that going from one domain to the other is rather difficult.